how to find resultant displacement|Component Addition (i.e., Analytical Method of Vector : Cebu This physics video tutorial explains how to find the magnitude and direction of the resultant displacement vector. This video also explains how to add vecto. Bovada's poker platform is also known for its lucrative tournaments, including daily and weekly events with substantial prize pools. For an immersive experience, Bovada's live dealer options bring the casino floor to your screen. Real dealers host games of blackjack, roulette, baccarat, and Super 6 in real-time, streamed in high definition .
how to find resultant displacement,This physics video tutorial explains how to find the magnitude and direction of the resultant displacement vector. This video also explains how to add vecto.how to find resultant displacementDisplacement Formula. Displacement is calculated as the shortest distance between starting and final point which prefers straight-line path over curved . Find resultant displacement in physics with help from a longtime experienced physics educator in this free video clip. Expert: Derek Hullinger Bio: Derek Hullinger received his PhD in physics.
The distance and displacement are both solved for in each problem using the pythagorean theorem, inverse trig functions, and vector addition. Each example shows the method of how you combine. The basic formula to calculate displacement is a reworking of the velocity formula: d = vt. Where d is displacement, v is average velocity, and t is the time period, or the .
Learn how to find the resultant of two or more vectors, such as displacement, velocity, or force. The resultant is the vector sum of the individual vectors and can be determined by vector .
how to find resultant displacement Component Addition (i.e., Analytical Method of Vector This follows from the Pythagorean theorem. The distance between two points P 1 with coordinates (x 1 , y 1 , z 1) and P 2 with coordinates (x 2, y 2, z 2) is. d = ( (x 2 - x 1) 2 + (y 2 - y 1) 2 + (z 2 - z1) 2)½. The distance d is the magnitude of the . The resultant displacement formula is given by relation $d=\sqrt{x^2+y^2}$ where, $d$ is displacement. $x$ is the object’s initial direction of motion and $y$ is the second direction in which the object moves.The direction of the resultant can be determined by finding the angle that the resultant makes with either the north-south or the east-west vector. The diagram at the right shows the angle theta (Θ) marked inside the vector . This physics video tutorial explains how to find the resultant of two vectors.Full 31 Minute Video on Patreon: https://www.patreon.com/MathScienceT.For example, we use this method in kinematics to find resultant displacement vectors and resultant velocity vectors, in mechanics to find resultant force vectors and the resultants of many derived vector quantities, and in electricity and magnetism to find resultant electric or magnetic vector fields.The resultant vector is the vector that 'results' from adding two or more vectors together. There are a two different ways to calculate the resultant vector. Methods for calculating a Resultant Vector: The head to tail method to .

The Independence of Perpendicular Motions. When we look at the three-dimensional equations for position and velocity written in unit vector notation, Equation 4.2 and Equation 4.5, we see the components of these equations are separate and unique functions of time that do not depend on one another.Motion along the x direction has no part of its motion along the y and z directions, .
Use the graphical technique for adding vectors to find the total displacement of a person who walks the following three paths (displacements) on a flat field. . The head-to-tail method outlined above will give a way to determine the magnitude and direction of the resultant displacement, denoted R R. Solution (1) Draw the three displacement .
Suppose, for example, that A is the vector representing the total displacement of the person walking in a city considered in Kinematics in Two Dimensions: . Find the components of the resultant along each axis by adding the components of the .Components along the same axis, say the x-axis, are vectors along the same line and, thus, can be added to one another like ordinary numbers.The same is true for components along the y-axis.(For example, a 9-block eastward walk could be taken in two legs, the first 3 blocks east and the second 6 blocks east, for a total of 9, because they are along the same direction.)Which indicates that the resultant force R has the same direction as a, and has magnitude equal to the product m a.. For example, if a box of 1.5 kg is subject to 5 forces which make it accelerate 2.0 m/s 2 north-west, then the resultant force is directed north-west and has the magnitude equal to 1.5 kg × 2.0 m/s 2 = 3.0 N.. Often, however, we know the forces that act on an object and . Resultant displacement formula. The resultant displacement formula is used when the distance from the point of reference is used to specify the initial and final position of the object. Despite the fact that distance and displacement are not the same things, displacement problems will tell you how many “foot” or “meters” an object has .

The maximum height is reached when \(\mathrm{v_y=0}\). Using this we can rearrange the velocity equation to find the time it will take for the object to reach maximum height \[\mathrm{t_h=\dfrac{u⋅\sin θ}{g}}\] where \(\mathrm{t_h}\) stands for the time it takes to reach maximum height. From the displacement equation we can find the maximum .
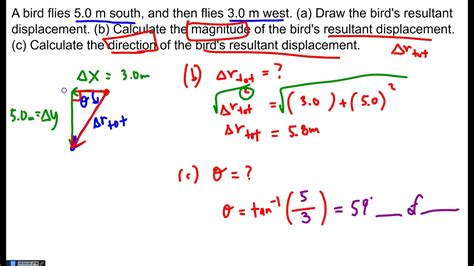
The result (or resultant) of walking 11 km north and 11 km east is a vector directed northeast as shown in the diagram to the right. Since the northward displacement and the eastward displacement are at right angles to each . In this video I go over an Example Problem dealing with the Physics of Vectors and show you how to find the Magnitude and Direction of Resultant Displacement.
Vector Addition: Head-to-Tail Method. The head-to-tail method is a graphical way to add vectors, described in Figure below and in the steps following. The tail of the vector is the starting point of the vector, and the head .
I review how to find the resultant graphically and then show how to do it algebraically. Suitable for high school physics.Khanmigo is now free for all US educators! Plan lessons, develop exit tickets, and so much more with our AI teaching assistant.
Component Addition (i.e., Analytical Method of Vector Example 1: Find the resultant of the vectors 4i + 3j -5k and 8i + 6j - 10k. Solution: The given two vectors are: A = 4i + 3j - 5k and B = 8i + 6j - 10k The direction ratios of the two vectors are in equal proportion and hence the two vectors are in the same direction. Resultant Vector. Vectors are entities that have a magnitude and a direction associated with them. For instance, velocity is a vector and will have a speed (magnitude) telling us how fast .
how to find resultant displacement|Component Addition (i.e., Analytical Method of Vector
PH0 · What is a Resultant?
PH1 · Resultant Displacement
PH2 · Position and displacement
PH3 · Physics Displacement Formula: How to Calculate Displacement
PH4 · How to find displacement in physics
PH5 · How to Solve Vector Problems (3 examples finding the Resultant
PH6 · How to Find Resultant Displacement in Physics
PH7 · How to Calculate Displacement (with Pictures)
PH8 · Displacement Formula with examples
PH9 · Component Addition (i.e., Analytical Method of Vector